Question

Find the potential function for f.

a) 1/(x⁶ y⁵) + C₁
b) -1/(x⁵ y⁶) + C₂
c) 1/(x⁵ y⁵) + C₃
d) -1/(x⁶ y⁶) + C₄

Answer 1

Main Answer:

The potential function for
`\(f\)` is given by the option:

c)
`\((1)/(x^5 y^5) + C_3\)`

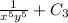
Step-by-step explanation:

The potential function
`\(F(x, y)\)` for a given function
`\(f(x, y)\)` is found by integrating each term with respect to its corresponding variable. In this case, if
`\(f(x, y)\) is \((1)/(x^5 y^5)\)`, the potential function
`\(F(x, y)\)` is obtained by integrating with respect to
`\(x\)` and
`\(y\)` separately.

`\[ F(x, y) = \int (1)/(x^5 y^5) \,dx = -(1)/(4x^4 y^5) + g(y) \]`

Now, we integrate
`\(g(y)\)` with respect to
`\(y\)`:

`\[ F(x, y) = \int -(1)/(4x^4 y^5) \,dy = (1)/(x^5 y^4) + C \]`

So, the correct option is c)
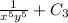`\((1)/(x^5 y^5) + C_3\)`, where
`\(C_3\)` is the constant of integration.