Final Answer:
The function has a removable discontinuity when
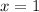 , and
, and
 can be used in the extended function to fill the hole.
can be used in the extended function to fill the hole.
Step-by-step explanation:
Analyzing the given function, we observe that it has points of discontinuity at
 , indicated by the provided values of
, indicated by the provided values of
 at these points. A removable discontinuity occurs when a function is not defined at a particular point but can be redefined at that point to make the function continuous. In this case, at
at these points. A removable discontinuity occurs when a function is not defined at a particular point but can be redefined at that point to make the function continuous. In this case, at
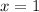 , there is a hole in the graph, as
, there is a hole in the graph, as
 is not provided. To fill this gap and make the function continuous at
is not provided. To fill this gap and make the function continuous at
 we use
we use
 as the value at this point. By assigning \(f(1) = 0\), we eliminate the removable discontinuity, and the function becomes continuous at
as the value at this point. By assigning \(f(1) = 0\), we eliminate the removable discontinuity, and the function becomes continuous at
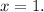
It's important to note that the other points of discontinuity at
 may not be removable, as the function might not be defined or may have different values at these points. Therefore, addressing each point of discontinuity individually is crucial to determine whether it is removable or essential for the overall continuity of the function.
may not be removable, as the function might not be defined or may have different values at these points. Therefore, addressing each point of discontinuity individually is crucial to determine whether it is removable or essential for the overall continuity of the function.