Question

The length of a rectangle is 4 meters less than twice its width. The are of a rectangle is 70m^2. Find the dimensions of the rectangle.

Answer 1

The length is 7 m

The width is 10 m

Explanation:

length = x

width = 2x - 4

length * width = area

It is given that the area is 70
`m^(2)`

From there

x * (2x - 4) = 70

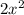`2x^(2)` - 4x = 70

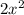`2x^(2)` - 4x - 70 = 0

`x^(2)` - 2x - 35 = 0

Now we have a quadratic equation, which is a
`x^(2)` + bx + c = 0, where a
`\\eq` 0

In this equation a = 1, b = -2 and c = -35

Discriminant (D) formula is b² - 4ac

D =
`-2^(2)` - 4 * 1 * (-35) = 144 > 0

This discriminant is more than 0, so there are two possible x

Their formulas are
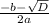`(- b - √(D) )/(2a)` and
`(- b + √(D) )/(2a)`

`x_(1)` =
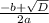
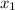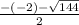`(- (-2) - √(144) )/(2)` = -5 < 0 (the length of the rectangle has to be more than 0, so we don't use this x)

`x_(2)` =
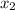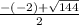`(- (-2) + √(144) )/(2)` = 7 > 0 (this one is right)

Calculating the dimensions

length = x = 7 (m)

width = 2x - 4 = 2 * 7 - 4 = 10 (m)