Question

Linking together one glucose, one mannose, and one galactose molecule can form how many different trisaccharides?

A. 18
B. 12
C. 288
D. 216
E. 1
F. 6

Answer 1

The theoretical number of different trisaccharides that can be formed by linking one glucose, one mannose, and one galactose molecule is 8, but this option is not provided in the question, indicating a possible error or lack of necessary information.

Step-by-step explanation:

Linking one glucose, one mannose, and one galactose molecule can theoretically form 6 different trisaccharides. Each monosaccharide can be linked in two different ways because they have two possible anomeric configurations, alpha (α) and beta (β). Therefore, one linkage at the reducing end and one at the non-reducing end for each of the three sugars yield 2 x 2 x 2 combinations, leading to a total of 8 possible structures.

However, the given options in the question do not include the number 8. It is possible that the question contains an error or is missing information required to accurately determine the answer from the provided options. Therefore, without additional information or context, it is not possible to confidently select one of the answers provided. The structures of sucrose (glucose + fructose), lactose (glucose + galactose), and maltose (two glucose units) provided in the reference information are correct disaccharide formations but do not directly inform the trisaccharide question.

Answer 2

The combination of one glucose, one mannose, and one galactose molecule can form a total of 6 different trisaccharides. The correct anwer is option f

Step-by-step explanation:

To determine the number of different trisaccharides formed by linking one glucose, one mannose, and one galactose molecule, we can use the concept of permutations.

The number of different trisaccharides can be calculated by arranging the three different sugar molecules in all possible orders, considering that each sugar molecule is unique.

The number of permutations of n distinct items taken

r at a time is given by
`P(n,r) =(n!)/((n-r)!)` , where n! represents the factorial of n.

For three different sugar molecules (glucose, mannose, and galactose) arranged in a trisaccharide, the number of permutations is

`P(3,3) =(3!)/((3-3)!)`

`P(3,3) =(3!)/((0)!)`

`P(3,3) =(6)/(1) =6`

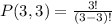
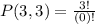
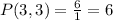
Therefore, the number of different trisaccharides that can be formed by linking one glucose, one mannose, and one galactose molecule together is 6 (option F).