The power is 5^(-3) because 125 is 5³ and negative exponents are reciprocals.
To solve this problem, we can use the following rule of exponents:
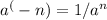
where a is any number and n is a positive integer.
Therefore, to find the power with a negative exponent that is equivalent to 1/125, we need to find the power of 125 that is equal to 1.
125^n = 1
Since 125 is 5 to the 3rd power, we can rewrite this equation as follows:
(5³)^n = 1
5^(3n) = 1
The only value of n that makes this equation true is n = -1.
5^(-3) = 1/5³ = 1/125
Therefore, the power with a negative exponent that is equivalent to 1/125 is
 .
.