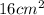Question

Solve for the area:



32 cm^2
16 cm
32 cm
16 cm^2

Answer 1

Given :

• Base of triangle = 8 cm
• Height of triangle = 4 cm

`\\`

To Find :

• Area of triangle = ?

`\\`

Solution :

As, we have :

• Base of triangle, b = 8 cm
• Height of triangle, h = 4 cm

`\\`

So, to find area of triangle we have a formula :

`\large \underline{\boxed{\bf{Area_((triangle)) = (1)/(2) * b * h}}}`

`\tt : \implies Area = (1)/(2) * 8 \: cm * 4 \: cm`

`\tt : \implies Area = (1)/(2) * 32 \: cm^2`

`\tt : \implies Area = \cancel{(32)/(2)} \: cm^2`

`\tt : \implies Area = 16 \: cm^2`

`\\`

Hence, area of given triangle is 16 cm².

Answer 2

The area of triangle ABC is
`16 cm^2`

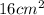
To find the area of triangle ABC, you can use the formula for the area of a triangle, which is 1/2 * base * height.

The area of triangle ABC is
`16 cm^2`

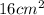
To find the area of triangle ABC, we can use the formula for the area of a triangle, which is 1/2 * base * height.

In this case, the base of the triangle is BC and the height is AM.

Since AM is perpendicular to BC and forms a right angle at M, AM is the height of the triangle.

Therefore, the area of triangle ABC is:

Area = 1/2 * BC * AM

Area = 1/2 * 8 cm * 4 cm

Area =
`16 cm^2`

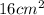
Therefore, the correct answer is B.
`16 cm^2`

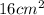
The probable question may be:

In triangle ABC, Side BC=8cm, a perpendicular line is drawn from point A to BC as AM=4cm, angle AMC=90 degree . Find the area of triangle.

A.
`32 cm^2`

B. 16 cm

C. 32 cm

D.
`16 cm^2`