Question

Mumuni Bawumia, a farmer sells 500 litres of fresh milk a day with a standard deviation of 35 litres. Given that the daily sales of fresh milk is normally distributed, what is the probability that the sales in any single day is:

a. Greater than 506 litres

b. Between 498 litres and 506 litres.
​

Answer 1

a: 0.43194

b: 0.09084

Explanation:

First construct the z values for 498 and 506.

`z=(x-u)/(s)`

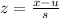
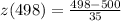`z(498)=(498-500)/(35)`=-0.05714

z(506)=(506-500)/35=0.17143

Check the cumulative normal distribution tables to find

P(x<=-0.05714)=0.47722

P(x<=506)=0.52278

These probabilities imply that

P(x>506)=1-P(x<=506)=1-0.52278=0.43194

P(498<=x<=506)=P(x<=506)-P(x<=498)=0.09084