Question

If n,6 M are in geometric sequence n,7.5, m are in arithmetic sequence ,then find the value of n and m?​

Answer 1

if n,6,m are in geometric sequence

=> 6/n = m/6

⇔ mn = 36 (1)

if n,7.5, m are in arithmetic sequence

=> 7.5 - n = m - 7.5

<=> m + n = 15 (2)

from (1)(2), we have the equations:

`\left \{ {{mn=36} \atop {m+n=15}} \right.\\\\<=>\left \{ {{mn=36} \atop {m=15-n}} \right.\\\\<=>\left \{ {{(15-n)n=36} \atop {m=15-n}} \right.\\\\<=>\left \{ {{n^(2)-15n+36 =0} \atop {m=15-n}} \right.\\\\<=>\left \{ {{n=12orn=3} \atop {m=15-n}} \right.`

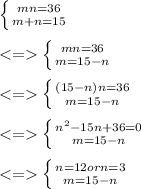
with n = 12 => m = 3

n = 3 => m = 12

=> (m;n) = {(3;12),(12;3)}

Explanation: