Question

169a2 - 52ab + 4b2
Factorise

Answer 1

(13a - 2b)²

Explanation:

169a² - 52ab + 4b² ← is a perfect square trinomial of the form

(ax - b)² = a²x² - 2abx + b²

Compare the coefficients of the squared terms

a² = 169 ⇒ a =
`√(169)` = 13

b² = 4 ⇒ b =
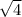`√(4 )` = 2

and - 2ab = - 2 × 13 × 2 = - 52 , then

169a² - 52ab + 4b² = (13a - 2b)² ← in factored form