Question

Suppose f(x) = x2 and g(x) = Which statement best compares the graph of g(x) with the graph of f(x)?​

Answer 1

g(x) is horizontally stretched by a factor of 1/5

Explanation:

Given

`f(x) = x^2`

`g(x) = ((x)/(5))^2`

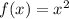
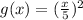
Require

Determine the relationship between f(x) and g(x)

`f(x) = x^2`

`g(x) = ((x)/(5))^2`

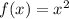
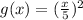
Express g(x) in terms of f(x)

`g(x)=f((x)/(5))`

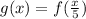
This can further be simplified as:

`g(x)=f((1)/(5)x)`

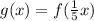
When a function is represented as:

`g(x) = f(cx)`

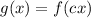
Where c < 1,

Then the type of translation is a horizontal stretch (stretch in the x direction)

By comparison:
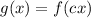`g(x) = f(cx)` and
`g(x)=f((1)/(5)x)`

`c = (1)/(5)`

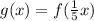
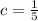
This means that: g(x) is horizontally stretched by a factor of 1/5