Question

A pharmacist found at the end of the day she had 3/2 as many prescriptions for antibiotics as tranquilizers. She had 35 prescriptions altogether. How many did she have for tranquilizers?

Answer 1

She had 14 prescriptions for tranquilizers.

Explanation:

This question can be solved using a system of equations.

I am going to say that:

x is the number of prescriptions she had for antibiotics.

y is the number of prescriptions she had for tranquilizers.

A pharmacist found at the end of the day she had 3/2 as many prescriptions for antibiotics as tranquilizers.

This means that:

`(x)/(y) = (3)/(2)`

`x = (3y)/(2)`

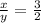
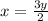
She had 35 prescriptions altogether.

This means that:

`x + y = 35`

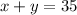
How many did she have for tranquilizers?

We want to find y. Since
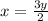`x = (3y)/(2)`, we solve the following equation.

`x + y = 35`

`(3y)/(2) + y = 35`

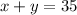
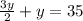
Multiplying everything by 2

`3y + 2y = 70`

`5y = 70`

`y = (70)/(5)`

`y = 14`

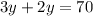
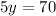
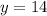
She had 14 prescriptions for tranquilizers.