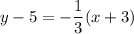Answer:
Point-slope form:
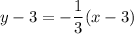
Slope-intercept form:
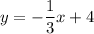
Explanation:
We want the equation in point-slope form and slope-intercept form that passes through the points (-3, 5) and (3, 3).
First, we will find the slope between the two points. By the slope formula:
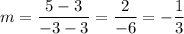
Point-slope form is given by:
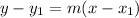
We can choose either point. I'm going to let (3, 3) be (x₁, y₁). The slope m is -1/3. Therefore:
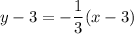
Is the point-slope form*.
To find the slope-intercept form, we simply need to isolate y. Distribute:
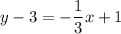
Adding 3 to both sides yields:
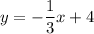
This is slope-intercept form.
*If you use the other point, the point-slope form will be: