Question

Find the surface area. Round to the hundredths place when necessary.

Question 2 options:

580 in²


980 in²


610 in²


715 in²

Answer 1

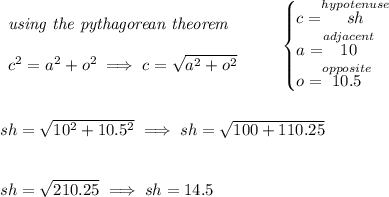
Check the picture below.

so let's firstly find the slant-height of the pyramid or namely "sh"

`\begin{array}{llll} \textit{using the pythagorean theorem} \\\\ c^2=a^2+o^2\implies c=√(a^2 + o^2) \end{array} \qquad \begin{cases} c=\stackrel{hypotenuse}{sh}\\ a=\stackrel{adjacent}{10}\\ o=\stackrel{opposite}{10.5} \end{cases} \\\\\\ sh=√( 10^2 + 10.5^2)\implies sh=√( 100 + 110.25 ) \\\\\\ sh=√( 210.25 )\implies sh=14.5`

so the area of the pyramid is really the area of four triangles whose altitude or height is 14.5 and with a base of 20, plus the area the 20x20 square on the base.

`\stackrel{ \textit{\LARGE Areas} }{\stackrel{\textit{four triangles}}{4\left[\cfrac{1}{2}(\underset{b}{20})(\underset{h}{14.5}) \right]}~~ + ~~\stackrel{ square }{(20)(20)}}\implies 580+400\implies \text{\LARGE 980}~in^2`