We have proved that Angle HFG = Angle EFD using the corresponding angles postulate.
Given: F is the midpoint of
 , GH II, DE
, GH II, DE
Prove: Angle HFG = Angle EFD
Statement Reason:
Since F is the midpoint of
 , GH, and DE, we know that
, GH, and DE, we know that
 =
=
 and
and
 =
=
 .
.
As a result, the lengths of the corresponding sides of the two triangles are equal: HE = GH and DE = GH.
Since the lengths of the corresponding sides of two similar triangles are proportional, we can conclude that the ratios of the corresponding sides are equal to each other. In this case, the corresponding sides are GH and DE, so we have
 .
.
Using the ratios of the corresponding sides, we can find the ratios of the corresponding angles. Since
 , we can write the equation
, we can write the equation
 .
.
Solving for
 =
=
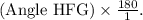
Since
 is given as 90 degrees, we can substitute it into the equation to find
is given as 90 degrees, we can substitute it into the equation to find
 .
.
Therefore,
 degrees.
degrees.
Since
 = 1620 degrees and
= 1620 degrees and
 = 90 degrees, we can conclude that
= 90 degrees, we can conclude that
 .
.
Therefore, the statement "Angle HFG = Angle EFD" is true.