Question

F(-5) = -4, and f(5)= 2
linear equation satisfying the conditions , if possible

Answer 1

Given:

f(-5) = -4, and f(5)= 2.

To find:

The linear equation satisfying the conditions.

Solution:

We have,

f(-5) = -4, and f(5)= 2

It means the function passes through the points (-5,-4) and (5,2). So, the linear equation of the function f is

`y-y_1=(y_2-y_1)/(x_2-x_2)(x-x_1)`

`y-(-4)=(2-(-4))/(5-(-5))(x-(-5))`

`y+4=(2+4)/(5+5)(x+5)`

`y+4=(6)/(10)(x+5)`

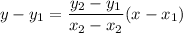
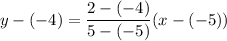
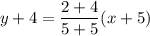
On further simplification, we get

`y+4=(3)/(5)(x+5)`

`y+4=(3)/(5)x+3`

`y=(3)/(5)x+3-4`

`y=(3)/(5)x-1`

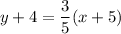
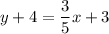
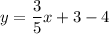
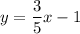
Putting y=f(x), we get

`f(x)=(3)/(5)x-1`

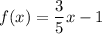
Therefore, the required function is
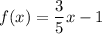`f(x)=(3)/(5)x-1`.