Question

Which is the graph of f(x) = (2)x

Answer 1

The graph of
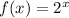`\( f(x) = 2^x \)` is an upward-sloping curve passing through (0,1) without touching the x-axis.

The function
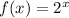`\( f(x) = 2^x \)`represents exponential growth. As x increases, the function value grows at an increasing rate. The graph of
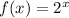`\( f(x) = 2^x \)` is an upward sloping curve that passes through the point (0,1) on the coordinate plane.

At x = 0, the function evaluates to
`\( f(0) = 2^0 = 1 \)`, so the graph crosses the y-axis at y = 1. As x increases, the function values grow rapidly because the base (2) raised to increasing powers results in exponential growth. This leads to a curve that rises steeply as x moves to the right.

The graph never touches the x-axis because 2 raised to any power (positive or negative) is never zero. However, as x approaches negative infinity, the function value gets closer and closer to zero, forming an asymptote along the x-axis.

The graph demonstrates the fundamental properties of exponential growth—rapid increase with positive x-values and approaching zero but never reaching it as x tends towards negative infinity.

The curve is continuous, smooth, and continuously increases without bound as x increases. This graphical representation showcases the growth pattern of exponential functions, illustrating their steep incline with positive x-values and their behaviour as x approaches negative infinity.