Answer:
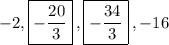

Explanation:
An arithmetic sequence is a sequence of numbers in which the difference between any two consecutive terms is constant.
The given terms of the sequence are:
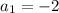
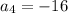
To find the common difference (d), we can divide the difference of the given terms by the difference of their positions:
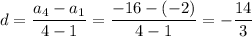
To find terms 2 and 3, add the common difference (d) to the previous term:
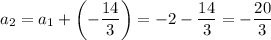
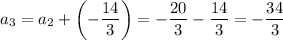
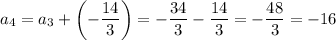
Therefore, the common difference of the given arithmetic sequence is -14/3, and the second and third terms are -20/3 and -34/3 respectively.