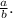To decompose
 into unit fractions, express it as
into unit fractions, express it as
 . This representation involves summing fractions with denominators matching the prime factors of 12, verifying the decomposition's accuracy.
. This representation involves summing fractions with denominators matching the prime factors of 12, verifying the decomposition's accuracy.
the fraction
 as an example.
as an example.
Steps to Decompose
 into Unit Fractions:
into Unit Fractions:
Factorize the Denominator:
Find the prime factorization of the denominator ( 12 ).
-
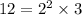
Express the Numerator as a Sum of Unit Fractions:
Express the numerator ( 7 ) as a sum of fractions using the prime factors found in step 1.
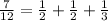
This expression is based on the factorization of 12:
 where 2 is repeated twice and 3 appears once.
where 2 is repeated twice and 3 appears once.
Verify the Result:
Sum up the unit fractions to confirm they equal the original fraction
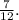

Final Result:
 can be decomposed into unit fractions as
can be decomposed into unit fractions as
 +
+
 . The sum of these unit fractions equals the original fraction.
. The sum of these unit fractions equals the original fraction.
complete the question
"How to Decompose Fractions into Unit Fractions?"
Decomposing fractions into unit fractions involves expressing a given fraction as a sum of fractions, where each fraction in the sum has a numerator of 1. To decompose a fraction like
 into unit fractions:
into unit fractions:
1. Find factors of the denominator
 .
.
2. Express the numerator
 as a sum of these unit fractions.
as a sum of these unit fractions.
3. Ensure that the sum of these unit fractions equals the original fraction