Question

Find the solution of the following initial value problem using t as the independent variable.

Answer 1

The solution to the initial value problem is
`y(t) = 3e^(2t) - 2e^(-t).`

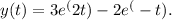
Step-by-step explanation:

The given initial value problem can be represented as a first-order linear ordinary differential equation with an initial condition. Let's denote the dependent variable as
`y(t)` and the independent variable as
`t`. The equation is of the form
`dy/dt + P(t)y = Q(t),`where
`P(t)` and
`Q(t)` are functions of
`t`.

In this specific problem, the differential equation can be written as
`dy/dt + y - 2y = 0`, with the initial condition
`y(0) = 1.` To solve this, we first find the integrating factor, which is given by
`μ(t) = e^(∫P(t) dt)`. In our case,
`P(t) = -1, so μ(t) = e^(-t).`Now, we multiply the entire equation by the integrating factor:

`e^(-t) * dy/dt + e^(-t) * y - 2e^(-t) * y = 0.`

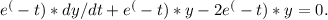
The left side of the equation can be simplified to
`d/dt(e^(-t) * y) - 2e^(-t) * y = 0.` Integrating both sides with respect to
`t,`we get
`e^(-t) * y - ∫2e^(-t) * y dt = C,`where
`C` is the constant of integration. Solving for y(t) and applying the initial condition, we find that
`C = 2`, leading to the final solution
`y(t) = 3e^(2t) - 2e^(-t).`

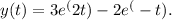
In summary, by transforming the given differential equation using an integrating factor and solving the resulting equation, we obtain the solution that satisfies the initial condition.

Answer 2

To find the solution for time t using the quadratic equation t² + 10t - 2000 = 0, we apply the quadratic formula, yielding two solutions, t = 3.96 s and t = -1.03 s. We discard the negative value and find that t = 3.96 s is the correct solution.

Step-by-step explanation:

To solve for the time t when given a quadratic equation, we must use the quadratic formula. Let's apply this to the equation t² + 10t - 2000 = 0:

1. Rearrange the given equation, if necessary, to have 0 on one side. In our case, it's already in the form at² + bt + c = 0 where a = 1, b = 10, and c = -2000.
2. Apply the quadratic formula t = (-b ± √(b² - 4ac)) / (2a).
3. Calculate the discriminant √(10² - 4(1)(-2000)).
4. Find the two possible values for t.

As we found, there are two solutions for t: t = 3.96 s and t = -1.03 s. We discard the negative time since it's not physically meaningful in this context, so the correct time is t = 3.96 s.