Question

Find the limit of the function lim t→0 3et - 3 t , 1 t - 1 t , 2 1 t?

Answer 1

The limit of the function as t approaches 0 is undefined.

Step-by-step explanation:

To find the limit of the function lim t→0 3et - 3 t , 1 t - 1 t , 2 1 t, we can simplify it as follows:

lim t→0
`3e^t - 3`

=
`3e^0 - 3`

= 3 - 3

= 0

`lim \ t\rightarrow0 (1/t - 1/t^2)`

=
`1/0 - 1/0^2`

=
`\infty - \infty`

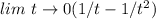
= undefined

`lim \ t\rightarrow 0 2/t`

=
`2/0`

=
`\infty`

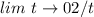
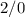
So, the limit of the function as t approaches 0 is undefined.