Question

The product of two consecutive negative integers is 20. Find the value of the lesser integer.​

Answer 1

The consecutive negative integers are -5 and -4. Their product, -5 * -4, equals 20. Therefore, the value of the lesser integer is -5.

Let the consecutive negative integers be \( x \) and \( (x-1) \). According to the problem, their product is 20, so we can set up the equation:

`\[ x \cdot (x-1) = 20 \]`

Expanding and rearranging the equation gives us a quadratic equation:

`\[ x^2 - x - 20 = 0 \]`

Now, we can factor the quadratic equation:

`\[ (x-5)(x+4) = 0 \]`

This implies that either
`\( x-5 = 0 \) or \( x+4 = 0 \).` Solving these equations gives us two possible values for
`\( x \): \( x = 5 \) or \( x = -4 \).` Since we're dealing with negative integers, we discard the positive solution, leaving us with
`\( x = -4 \).`

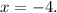
Therefore, the consecutive negative integers are
`\( -4 \)` and
`\( -5 \)`, and the lesser integer is
`\( -5 \).` So, the answer to the problem is that the value of the lesser integer is
`\( -5 \).`