Question

the motion of a body described by the equation. S(t) = 25t+6t² what is the velocity instantaneous at t = 2 second​

Answer 1

49 units per seconds

Step-by-step explanation:

To find the instantaneous velocity of a body described by the position function S(t) = 25t + 6t² at a specific time (t seconds), we need to find the velocity function v(t) by differentiating the position function with respect to time (t).

To differentiate S(t), we can use the power rule for differentiation:

`\boxed{\begin{array}{c}\underline{\textsf{Power Rule for Differentiation}}\\\\\frac{\text{d}}{\text{d}x}\left(x^n\right)=nx^(n-1)\\\end{array}}`

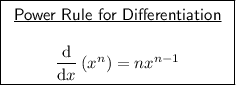
Therefore:

`\begin{aligned}v(t)=\frac{\text{d}S}{\text{d}t}&=\frac{\text{d}}{\text{d}t}(25t+6t^2)\\\\&=1\cdot 25t^(1-1)+2 \cdot 6t^(2-1)\\\\&=25+12t\end{aligned}`

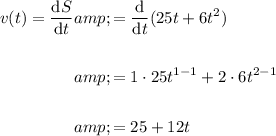
Now, to find the instantaneous velocity at t = 2 seconds, substitute t = 2 into the velocity function:

`\begin{aligned}v(2) &= 25 + 12(2)\\\\v(2)&=25+24\\\\v(2)&=49\end{aligned}`

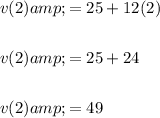
Therefore, the instantaneous velocity of the body at t = 2 seconds is 49 units per seconds.