Answer:
2s [seconds], 6s [seconds]
Step-by-step explanation:
Using the equations of Motion :-
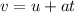
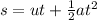
We pick our best formula for our need
--
NOTE:: ACCELERATION (A) = GRAVITY = 9.8 MS-2 OR 49/5 MS-2
NOTE:: ''•''MEANS MULTIPLICATION AND ''^'' MEANS POWER OF..
NOTE:: TRANSPOSE MEANS TO SWITCH SIDES IN LINEAR EQUATION & + goes -, - goes + etc
--
Inital Velocity (u) = 29.4 ms-1
Displacement (s) = 34.3m
--
1)
- v = u + at OR v = u + gt
- v [velocity not given] = 0, u = 29.4, g = -9.8 [approx on earth {negative because gravity is pulling down}] and we need to find t
- 0 + 29.4 + -9.8 • t
- 0 + 29.4 + -9.8t [transpose -9.8t]
- 9.8t = 29.4
- t = 29.4/9.8
- t = 3
- 3s
.. 3s [seconds]
2)
- s = ut+1/2at^2 OR s = ut + 1/2gt^2
- s [displacement is 0m as when it reaches ground level, displacement/height would be 0m] = 0m, g = -9.8 ms-2, u = 29.4 ms-1, and we need to find t
- 0 = 29.4 • t + 1/2 • -9.8 • t^2
- 0 = 29.4t + (-9.8t^2)/2 [transpose (-9.8t^2)/2 and solve (-9.8t^2)/2]
- 4.9t^2 = 29.4t
- t^2 = (29.4t)/4.9
- t^2 = 6t
- (t^2)/t = 6 • t/t
- t = 6
- 6s
.. 6s [seconds]