The function f(x) should be matched with their respective inverse function as follows;
A. f(x) = 5x + 10 ↔ 1.

B. f(x) = 15x - 6 ↔ 3.
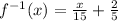
C. f(x) = 15x + 10 ↔ 2.
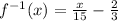
In Geometry, an inverse function is a type of function that is obtained by reversing the mathematical operation in a given function (f(x)).
In this exercise, we have to determine the inverse of the square function f(x). This ultimately implies that, we would have to interchange both the independent value (x-value) and dependent value (y-value) as follows;
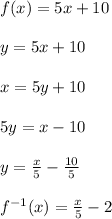
Part B.
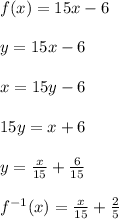
Part C.
In this context, we can logically deduce function C must be matched to the second inverse function.