CD=18 and CE=18.
The centroid of a triangle is a point of concurrency of its medians. The medians divide each other into segments with a ratio of 2:1, where the longer segment is closer to the vertex. Let's consider triangle
ABC with centroid D.
Given that
DE=9 and D is the centroid, we can use the median property to find CD and CE.
Since DE is one-third of the median from vertex A, we can express
AD as 3×DE:
AD=3×DE=3×9=27
Now, since CD and CE are the other two medians, we can express them in terms of AD as follows:
CD =
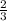 AD
AD
CD =
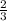 x 27 = 18
x 27 = 18
CE =
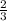 AD
AD
CE = =
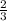 x 27 = 18
x 27 = 18
Therefore, CD=18 and CE=18.