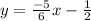Question

Write the equation of the line perpendicular to y = 6/5x + 4/5 that passes through the point (-3,2).

Answer 1

The equation of the perpendicular line of the given line is

`y = (-5)/(6) x -(1)/(2)`

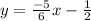
Explanation:

Step(i):-

Given the equation of the line

`y = (6)/(5) x + (4)/(5)`

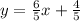
cross-multiplication, we get

5y = 6x +4

The equation of the straight line is 6x - 5y +4=0

The equation of the perpendicular line to the given line is

b x - ay +k=0

The equation of the perpendicular line to the given line is -5x-6y +k=0

This line passes through the point (-3,2)

⇒ - 5(-3) -6(2)+k=0

⇒ 15 -12 +k=0

⇒ 3 +k=0

⇒ k =-3

Step(ii):-

The equation of the perpendicular line is

-5x-6y-3=0

5x + 6y +3 =0

6y = -5x-3

`y = (-5)/(6) x -(3)/(6)`

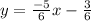
Final answer:-

The equation of the perpendicular line of the given line is

`y = (-5)/(6) x -(1)/(2)`