Question

Pentagon A’B′C′D′E’, is the image of pentagon ABCDE under a dilation with a scale factor of 1/2

Answer 1

The length of AE is
`A'E' * (2)/(3)`.

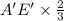The relationship between corresponding sides of similar figures under dilation is based on the scale factor. Here, the scale factor is
`(3)/(2)`

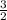
If Pentagon A′ B′ C′ D′E′ is the image of Pentagon ABCDE under this dilation, the length of a side of the original pentagon

ABCDE corresponds to
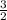`(3)/(2)` times the length of the corresponding side in the image A′ B′ C′ D′E′

Given that

AE is a side of the original pentagon, and the scale factor is
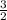`(3)/(2)` , the length of A'E' in the image will be
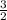`(3)/(2)`​ times the length of AE. Therefore, to find the length of AE, you can divide the length of A′E' by the scale factor
`(3)/(2)`

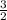
​If you have the length of A′E′ , you can find the length of AE by using the formula:

AE=
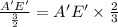
`(A'E')/((3)/(2) ) =A'E' * (2)/(3)`

AE =
`A'E' * (2)/(3)`

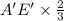
​So, The length of AE is
`A'E' * (2)/(3)`.

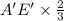Question

" Pentagon A’B′C′D′E’ Is the image of pentagon ABCDE under a dilation with a scale factor of 3/2 What is the length of segment overline AE ?"--