Question

Five clowns are late to a party they jump into their sporty coupe and start driving. Eventually they come to a level curve, with a radius of 27.5. What is the top speed at which they can drive successfully around the curve? The coefficient of friction between the car’s tires and the road is .8

Answer 1

The top speed at which the five clowns can successfully drive around the curve is approximately 15.21 m/s.

Step-by-step explanation:

To calculate the top speed at which the five clowns can successfully drive around the curve, we need to use the formula for centripetal force:

`Fc = mv^2/r`

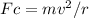
Where Fc is the centripetal force, m is the mass, v is the speed, and r is the radius of the curve.

We know the radius is 27.5 m, and the coefficient of friction is 0.8. The maximum frictional force can be calculated using the formula:

fmax = µN

Where fmax is the maximum frictional force, µ is the coefficient of friction, and N is the normal force. The normal force can be calculated using the equation:

N = mg

Where m is the mass of the car and g is the acceleration due to gravity.

By equating the centripetal force and maximum frictional force, we can solve for the maximum speed:

`mv^2/r = µmg`

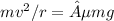
Simplifying the equation gives:

v = sqrt(µgr)

Plugging in the values, we get:

v = sqrt(0.8 * 9.8 * 27.5)

v ≈ 15.21 m/s