Question

5) If a projectile on Earth is fired straight upward so the distance in feet above the ground in t seconds

after firing is given by d (t) = -16t² + 400t. When will the projectile reach a height of 250 feet?

Answer 1

We are looking for the value of t when d(t) equals 250. So:

`d(t)=250\\-16t^2+400t=250`

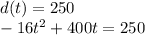
So let's solve for t. The best way to do this is by using the Quadratic Formula. This evaluates quadratic equations. Quadratic equations are trinomials with a degree of two.

`x=(-b\pm√(b^2-4ac))/(2a)\\\\-16t^2+400t=250\\-16t^2+400t-250=250-250\\-16t^2+400t-250=0\\\\t=(-400\pm√(400^2-4(-16)(-250)))/(2(-16))\\t=(-400\pm√(160000-4(4000)))/(-32)\\t=(-400\pm√(160000-16000))/(-32)\\t=(-400\pm√(144000))/(-32)\\t=(-400\pm379.47)/(-32)\\t=(-20.53)/(-32),(-779.47)/(-32)\\t=0.641,24.36`

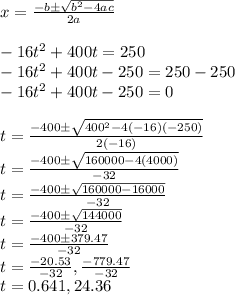
So the projectile will be at a height of 250ft at 0.641 seconds and 24.36 seconds.