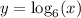Question

P

-8
6
+
1-3
0.5, -0.4) (1.0)
7 7 9 9
4
Y
-10
2
y = log1x
y = logo.5 X
y = log₁x
y = log, x
4
6
100
8
X

Answer 1

The equation of the function represented by the graph is
`y = \log_6(x)`

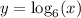
How to determine the equation of the function

From the question, we have the following parameters that can be used in our computation:

The graph

A logarithmic function is represented as

`y = \log_b(x)`

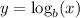
Where, b is the base of the function

Using the point (6, 1), we have

`\log_b(6) = 1`

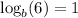
So, we have

`6 = b^1`

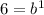
Evaluate

b = 6

Recall that

`y = \log_b(x)`

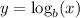
So, we have

`y = \log_6(x)`

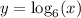
Hence, the equation of the function is
`y = \log_6(x)`