Question

A stadium has 55,000 seats. Seats sell for ​$28 in Section​ A, ​$16 in Section​ B, and ​$12 in Section C. The number of seats in Section A equals the total number of seats in Sections B and C. Suppose the stadium takes in ​$1,156,400 from each​ sold-out event. How many seats does each section​ hold?

Answer 1

Answer: Section A holds 27500 , Section B has 14100 and SEction C has 13400.

Explanation:

Let x= Number of seats in section A.

y = Number of seats in section B.

z= NUmber of seats in sectionC.

As per given , we have

x+y+z=55000 (i)

x= y+z (ii)

28x+16y+12z=1156400 (iii)

Substitute value of x from (ii) in (i), we get

`x+x=55000\\\\\Rightarrow\ 2x=55000\\\\\Rightarrow\ x=27500`

From (i)

`27500+y+z=55000\\\\\Rightarrow\ y+z=55000-27500\\\\\Rightarrow\ y+z=27500 \ \ \ (iv)`

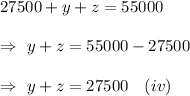
From (iii), we get

`28(27500)+16y+12z=1156400 \\\\\Rightarrow\ 770000+16y+12z=1156400\\\\\Rightarrow\ 16y+12z=386400\\\\\Rightarrow\ 4y+3z=96600 \ \ \ \ (v)`

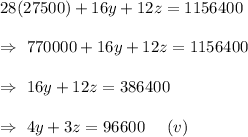
Multiply 3 to (iv), we get

`3y+3z=82500\ \ \ \ (vi)`

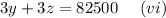
Eliminate (v) from (iv), we get

`y=14100`

from (iv)

`14100+z=27500\\\\\Rightarrow\ z=13400`

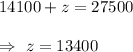
Hence, Section A holds 27500 , Section B has 14100 and SEction C has 13400.