Question

What is the tangential velocity of erth if the radius is 6400km and the period is 24hr ?

Answer 1

465.4 m/s (rounded to 1 d.p)

Step-by-step explanation:

To determine the tangential velocity of the Earth given its radius and period, we'll use the formula for calculating the tangential velocity in circular motion, which is given by:

`v = (d)/(t) =\boxed{(2\pi r)/(t)}`

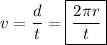
Where:

• 'v' is the tangential velocity (our unknown)
• 'r' is the radius of the Earth
• 't' is the time it takes the Earth to spin one revolution

Given:

• r = 6400 km
• t = 24 hr

Solve by substituting in our given information:

`\Longrightarrow v = \frac{2\pi \left(6400 \text{ km } * \frac{1000 \text{ m}}{1 \text{ km}}\right)}{\left(24 \text{ hr } * \frac{3600 \text{ s}}{1 \text{ hr}}\right)}\\\\\\\\\Longrightarrow v = \frac{2\pi \left(6400000 \text{ m}\right)}{86400 \text{ s}}\\\\\\\\\Longrightarrow v = \frac{4000\pi \text{ m}}{27 \text{ s}}\\\\\\\\\therefore v \approx \boxed{465.4 \ \text{m/s}}`

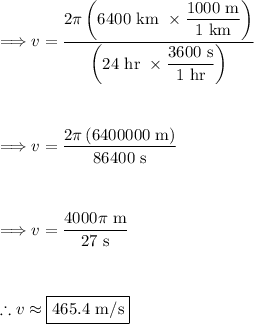
Thus, the tangential velocity of the Earth is approximately 465.4 meters per second.