p, q, and r indicate placeholders (aka variables) for true or false statements. We denote those as T and F respectively.
Since we have 3 variables and 2 choices each, there are 2^3 = 8 different combinations of T and F.
Refer to the table below to see all of the combinations. The first column p has four T's and then four F's. The next column q has TT followed by FF and then that pattern is repeated. The third column r will have T and F repeated until getting 8 total (4 of each).

Another way to form that table is to use the binary number system. Treat 0 as F and 1 as T. Or we could easily flip things around to have 0 represent T and 1 represent F.
From there, we form the q ^ r column. This is where we "and" together columns q and r. This is known as a logical conjunction. Recall that the result of "and" is true only when both parts are true; otherwise, the result is false.
With that in mind, you should get this new column shown below.

We'll then apply a boolean "or" operation with that column we just formed, and the p column. The result of an "or" is false only when both parts are false; otherwise it is true. It's basically the opposite of "and" (more or less).
Here's the fully completed table.
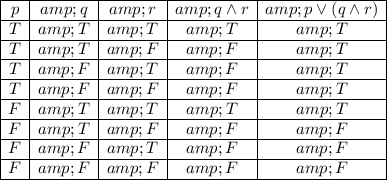
The most important column is the last column of course, but it depends on the previously columns formed earlier. I suppose if you wanted you could erase the q ^ r column and still have a coherent truth table. Personally I'd keep it in since it helps show the teacher your scratch work.
A spreadsheet is useful to help organize everything.