Question

Given the graph of the logarithmic function below, determine the equation in the form f(x) = k + logbx.

b=?
k=?

Answer 1

`f(x)=1+log_(2)(x)`

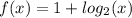
Explanation:

The given function is
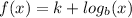`f(x)=k+log_(b)(x)`, and we know some points such that f passes through them, like (1,1) and (2,2). then we can get the next equations:

`f(1)=1=k+log_(b)(1)\\`

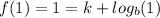
but
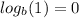`log_b(1)=0\\` given that
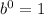`b^0=1` for any number b, then:

`1=k+0=k`

Similar, we can take the point (2,2):

`2=f(2)=1+log_b(2)\\1=log_b(2)\\b^1=2\\b=2\\`

The last equalities are given by definition of logarithm