Question

Which equation is represented by the graph below?

Answer 1

`y=-(1)/(2)x+2`

Explanation:

To find the slope, you need to put it in the form of y=mx+b. M is the slope, and b is the y intercept.

First find the slope.

To do this arbitrarily choose any two points on the line. Point 1 (0, 2) and Point 2 (4, 0)

To find the slope(m) calculate (difference in y)/(difference in x) or
`(rise)/(run)`, so use the formula which is
`m=(y_(2)-y_(1) )/(x_(2)-x_(1))`.

`y_(2)` is the y coordinate in point 2,
`y_(1)` is the y coordinate in point 1.

`x_(2)` is the x coordinate in point 2,
`x_(1)` is the x coordinate in point 1.

So put the proper numbers into the formula.

`m=(0-2)/(4-0)` And simplify.

m =
`(-2)/(4)`

m =
`(-1)/(2)`

m =
`-(1)/(2)`

So y=
`-(1)/(2)` x+b

Next to find b, choose a point and put in the x and y coordinates.

(0, 2)

2=-1/2(0)+b

Simplify

2=b

b=2

Now find the final equation of the line

y=-1/2 x +2

Answer 2

`\sf y = -(1)/(2)x + 2`

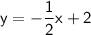
Explanation:

To find the equation of the line in a graph.

Let's take two points:

(0,2) and (4,0)

Now, we can use the slope-intercept form of a linear equation, which is given by:

`\sf y = mx + b`

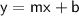
where:

-
`\sf m` is the slope of the line, and

-
`\sf b` is the y-intercept (the y-coordinate of the point where the line intersects the y-axis).

The slope (
`\sf m`) can be found using the formula:

`\sf m = \frac{{\textsf{{change in }} y}}{{\textsf{{change in }} x}}`

Given the points (0,2) and (4,0), we can calculate the slope:

`\sf m = \frac{{0 - 2}}{{4 - 0}}`

`\sf m = \frac{{-2}}{{4}}`

`\sf m = -(1)/(2)`

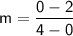
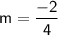
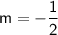
Now that we have the slope
`\sf (m)`, we can use one of the points to find the y-intercept (
`\sf b`). Let's use the point (0,2):

`\sf 2 = (-(1)/(2)) \cdot 0 + b`

`\sf 2 = b`

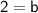
Now that we have both
`\sf m` and
`\sf b`, you can write the equation of the line:

`\sf y = -(1)/(2)x + 2`

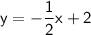
So, the equation of the straight line is
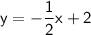`\sf y = -(1)/(2)x + 2`.