Question

There are 45 swimmers in the Top Swim club.

All swimmers are learning butterfly and backstroke and are asked
which they prefer.
of all swimmers prefer backstroke.
The number of juniors is double the number of seniors in the club.
of the juniors prefer butterfly.
Work out the proportion of swimmers who are seniors and prefer
backstroke.
You may use the table to help you.
Seniors
Juniors
Total
(5 marks)
Prefer to swim
Butterfly Backstroke Total
Proportion
45

Answer 1

`\begin{array}c\cline{1-4}&\sf Butterfly&\sf Backstroke&\sf Total\\\cline{1-4}\sf Seniors&13&2&15\\\cline{1-4}\sf Juniors&5&25&30\\\cline{1-4}\sf Total&18&27&45\\\cline{1-4}\end{array}`

`\textsf{Proportion of swimmers who are seniors and prefer backstroke}=(2)/(45)`

Explanation:

There are a total of 45 swimmers in the club.

If 3/5​ of all swimmers prefer backstroke, we can calculate the total number of swimmers who prefer backstroke by multiplying this proportion by the total number of swimmers:

`\textsf{Total backstroke}=(3)/(5) * 45 = (135)/(5)=27`

Now, we can calculate the total number of swimmers who prefer butterfly by subtracting the total number of swimmers who prefer backstroke (27) from the total number of swimmers (45):

`\textsf{Total butterfly}=45-27=18`

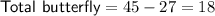
Let's denote the number of juniors in the club as J, and the number of seniors in the club as S. Given that there a total of 45 swimmers in the club, and the number of juniors is double the number of seniors, we can create two equations:

`S+J=45`

`J=2S`

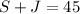
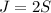
To solve this system of equations, substitute J = 2S into S + J = 45 and solve for the number of seniors (S):

`S + 2S = 45 \implies 3S=45 \implies S=(45)/(3)=15`

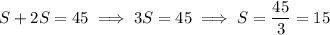
Therefore the total number of seniors is 15.

To find the number of juniors, substitute S = 15 into J = 2S:

`J=2(15)=30`

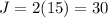
Therefore, the total number of juniors is 30.

Given that 1/6 of the juniors prefer butterfly, we can calculate the number of juniors who prefer butterfly by multiplying this proportion by the total number of juniors (30):

`\textsf{Juniors butterfly}=(1)/(6) * 30=(30)/(6)=5`

Therefore, after entering the values into the provided table, we get:

`\begin{array}c\cline{1-4}&\sf Butterfly&\sf Backstroke&\sf Total\\\cline{1-4}\sf Seniors&&&15\\\cline{1-4}\sf Juniors&5&&30\\\cline{1-4}\sf Total&18&27&45\\\cline{1-4}\end{array}`

To calculate the number of seniors who prefer butterfly, subtract the number of juniors who prefer butterfly (5) from the total number of swimmers who prefer butterfly (18):

`\textsf{Seniors butterfly}=18-5=13`

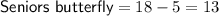
To calculate the number of juniors who prefer backstroke, subtract the number of juniors who prefer butterfly (5) from the total number of juniors (30):

`\textsf{Juniors backstroke}=30-5=25`

`\begin{array}c\cline{1-4}&\sf Butterfly&\sf Backstroke&\sf Total\\\cline{1-4}\sf Seniors&13&&15\\\cline{1-4}\sf Juniors&5&25&30\\\cline{1-4}\sf Total&18&27&45\\\cline{1-4}\end{array}`

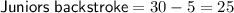
Finally, to calculate the number of seniors who prefer backstroke, subtract the number of seniors who prefer butterfly (13) from the total number of seniors (15):

`\textsf{Seniors backstroke}=15-13=2`

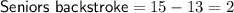
Therefore, the completed table is:

`\begin{array}c\cline{1-4}&\sf Butterfly&\sf Backstroke&\sf Total\\\cline{1-4}\sf Seniors&13&2&15\\\cline{1-4}\sf Juniors&5&25&30\\\cline{1-4}\sf Total&18&27&45\\\cline{1-4}\end{array}`

From observation of the table, the number of swimmers who are seniors and prefer backstroke is 2. Therefore, the proportion of swimmers who are seniors and prefer backstroke can be calculated by dividing this number by the total number of swimmers:

`\textsf{Seniors and backstroke}=(2)/(45)`