Question

If the first term and the common ratio of GPA are - 7 and 1/2 respectively, then find 10th term​

Answer 1

`\sf a_(10) = -(7)/(512)`

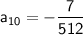
Explanation:

In a geometric progression (G.P.), the
`\sf n`th term (
`\sf a_n`) is given by the formula:

`\sf a_n = a \cdot r^((n-1))`

where:

-
`\sf a` is the first term,

-
`\sf r` is the common ratio, and

-
`\sf n` is the term number.

Given that the first term
`\sf a = -7` and the common ratio
`\sf r = (1)/(2)`, and we want to find the 10th term (
`\sf a_(10)`), we can substitute these values into the formula:

`\sf a_(10) = -7 \cdot \left((1)/(2)\right)^9`

Now, calculate this value:

`\sf a_(10) = -7 \cdot (1)/(512)`

`\sf a_(10) = -(7)/(512)`

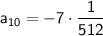
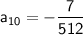
Therefore, the 10th term of the given geometric progression is
`\sf -(7)/(512)`.