Question

`y=a\left(b\right)^{x}`

Answer 1

The expression
`y=a(b)^x` represents an exponential function, where constants a and b, and variables x and y indicate the rate of growth or decay as x varies.

The expression
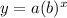`y=a(b)^(x)` represents an exponential function, where a and b are constant numbers, and x is the independent variable while y is the dependent variable. In such functions, the variable x appears as an exponent, and that is why it is called 'exponential.'

The base b is a positive number, and it determines the rate at which the function grows or decays. The coefficient a affects the steepness and direction of the graph.

If a student is given an expression like
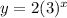`y=2(3)^(x)`, to find the value of y when x is 1, they would substitute 1 for x to get
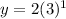`y=2(3)^(1)`, which simplifies to y=2*3, resulting in y=6.

The probable question may be: " What does the expression
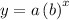`y=a\left(b\right)^(x)` represent"