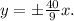Question

A hyperbola centered at the origin has a vertex at (0,-40) and a focus at (0,41)

which are the equations of the asymptotes?

Answer 1

For a hyperbola with a vertical transverse axis centered at the origin, vertex (0, -40), and focus (0, 41), the equations of the asymptotes are
`\(y = \pm(40)/(9)x\).`

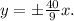
A hyperbola centered at the origin with a vertex at (0, -40) and a focus at (0, 41) is characterized by a vertical transverse axis. The standard form equation for such a hyperbola is given by
`\((y^2)/(a^2) - (x^2)/(b^2) = 1\)`, where a is the distance from the center to the vertices, and \(b\) is the distance from the center to the foci.

In this case, since the hyperbola is centered at the origin, the vertex coordinates (0, -40) provide the value of a, which is 40. The distance from the center to the focus, c, is determined by the Pythagorean relationship c^2 = a^2 + b^2. Given that the focus is at (0, 41), c is found to be 41. Substituting these values into the equation, we get b^2 = c^2 - a^2, resulting in b^2 = 41^2 - 40^2.

The standard form equation becomes
`\((y^2)/(1600) - (x^2)/(81) = 1\)`. The asymptotes for a hyperbola centered at the origin with a vertical transverse axis are given by the equations
`\(y = \pm(a)/(b)x\)`, which, in this case, lead to the asymptotes
`\(y = \pm(40)/(9)x\).`