The line
 does not intersect the circle
does not intersect the circle
 when
when
 or
or
 , as determined by the discriminant of the quadratic equation derived from the circle.
, as determined by the discriminant of the quadratic equation derived from the circle.
To find the values of
 for which the line
for which the line
 does not intersect the circle with equation
does not intersect the circle with equation
 , we need to substitute
, we need to substitute
 into the circle equation and solve for
into the circle equation and solve for
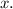
The circle equation is
 Substitute
Substitute
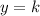
![\[ x^2 + k^2 - 2x - 3k - 4 = 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/bxfqpmqjteyeikzcv9kx52fqt77ma3eil5.png)
Now, rearrange the terms and express it as a quadratic equation:
![\[ x^2 - 2x + k^2 - 3k - 4 = 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/ryg54uayv9f3o87s6ko6v1w78imrps80zc.png)
For the line
 not to intersect the circle, the discriminant of this quadratic equation
not to intersect the circle, the discriminant of this quadratic equation
 must be negative.
must be negative.
The discriminant is given by
 :
:
![\[ \Delta = 4 - 4(k^2 - 3k - 4) \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/6u52mfdf2gc6aqhamj9fn5vcud89c0g6sc.png)
Expand and simplify:
![\[ \Delta = 4 - 4k^2 + 12k + 16 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/w9u7j5ke2m655tkqlcxzxmsf8ayah5sd0r.png)
Now, set the discriminant less than zero:
![\[ 4 - 4k^2 + 12k + 16 < 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qqmuqwqqma9ytttf1vgv39jt5kvaaq18xi.png)
Combine like terms:
![\[ -4k^2 + 12k + 20 < 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/lti9mud3v4ofvr1wvqkm9fufwa6bpmltuj.png)
Divide by -4 to simplify:
![\[ k^2 - 3k - 5 > 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/6c4d9n1k6bdxo342h3kq7gsh50jliuaqep.png)
Now, factor the quadratic expression:
![\[ (k - 5)(k + 1) > 0 \]](https://img.qammunity.org/2024/formulas/mathematics/high-school/rozokb8dp1c0gwepyf0rn1tqh72p8g6kg8.png)
The critical points are
 and
and
 Test intervals to determine when the expression is positive:
Test intervals to determine when the expression is positive:
- When
 Both factors are negative, so the expression is positive.
Both factors are negative, so the expression is positive.
- When
 : The factor
: The factor
 is positive, and
is positive, and
 is negative, so the expression is negative.
is negative, so the expression is negative.
- When
 : Both factors are positive, so the expression is positive.
: Both factors are positive, so the expression is positive.
Therefore, the values of
 for which the line
for which the line
 does not intersect the circle are
does not intersect the circle are
