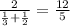Question

The latus rectum of a parabola whose focal chord is PSQ such that SP=3 and SQ=2, is given by

a. 24/5
b. 12/5
c. 6/5
d. 48/5

Answer 1

The latus rectum of the parabola is 24/5.

The latus rectum of a parabola is the distance between the two points on the parabola that are farthest from the directrix. The semi-latus rectum is half of this distance. The semi-latus rectum is also equal to the harmonic mean of the two segments of any focal chord of the parabola.

The harmonic mean of two numbers is defined as the reciprocal of the average of their reciprocals. In other words, the harmonic mean of two numbers a and b is:

`(2)/((1)/(a) + (1)/(b))`

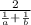
In this case, the harmonic mean of SP and SQ is:

`(2)/((1)/(3) + (1)/(2)) = (12)/(5)`