Final Answer:
The rational expressions matched to their simplest forms are:
(x² - 4) / (x² - 2x - 8) = (x + 2) / (x - 4)
(3x² + 6x) / (3x² - 12) = (x + 2) / (x - 4)
(5x² - 25x) / (20x² - 5) = (x - 5) / (4x + 1)
Step-by-step explanation:
In the first rational expression, we factorize the numerator and denominator to get (x + 2)(x - 2) / (x + 2)(x - 4). We then cancel out the common factor of (x + 2), leaving us with the simplest form of (x - 2) / (x - 4).
For the second expression, we factor out a common factor of 3x from the numerator and denominator, resulting in (3x(x + 2)) / (3(x² - 4)). We simplify further by canceling out the common factor of 3, giving us the simplest form of (x + 2) / (x - 4).
Lastly, in the third expression, we factor out a common factor of 5x from the numerator and a common factor of 5 from the denominator, leading to x(x - 5) / 5(4x + 1). This simplifies to the simplest form of (x - 5) / (4x + 1).
These simplifications are achieved through factoring and canceling out common factors, resulting in the expressions being represented in their simplest forms.
Complete Question:
Given the rational expressions matched to their simplest forms:
1.

2.
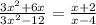
3.
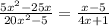
Explain the simplification process for each expression, including the factoring steps and the cancellation of common factors. Illustrate how the original expressions are transformed into their simplest forms.