Question

The joint distribution for the lifetimes of two different types of components operating in a system is given by

f(x,y)={1/8 ​xeˣ⁺ʸ/²​, if x,y > 0
{0, otherwise. ​

Find the density function for the ratio W=Y​/X.

Answer 1

The density function for the ratio of the lifetimes of the two types of components is
`(5ue^(-w))/(w^2)`, where w is the ratio of the lifetime of the second component to the first.

The joint density function for the lifetimes of two different types of components operating in a system is given by:

f(x,y)={
`10xe^(-(x+y))` , if x,y>0,

0, otherwise.

​We are asked to find the density function for the ratio

W= y/x

To do this, we can use the transformation technique. Let's define two new random variables:

U=X+Y

V=Y−X

The Jacobian of this transformation is:

`J = \left| \begin{pmatrix} (\partial U)/(\partial x) & (\partial U)/(\partial y) \\ \\ (\partial V)/(\partial x) & (\partial V)/(\partial y) \end{pmatrix} \right| = 1`

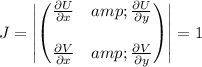
We can now relate the joint density function of U and V to the joint density function of X and Y using the transformation rule:

`f_U`,V(u,v) =
`f _X` ,Y(x,y)∣J∣

We can rewrite x and y in terms of u and v using the definition of U and V:

x = u−v / 2

​y= u+v / 2

​Substituting into the joint density function of X and Y, we get:

`f_U`,V(u,v) = 10( u−v / 2)
`e^{(u-v)/(2) + (u+v)/(2)}` |1|

Simplifying, we get:

`f_U` ,W(u,v)=5u
`e^ {-u}`for u>v

We are interested in the density function for W, which is V/U. To find this, we need to perform another variable transformation. Let W= V/U. Then, V=UW. Substituting into the joint density function of U and V, we get:

`f_U` ,W(u,w)=5u
`e^ {-u}` |
`J_W`|

The Jacobian of this transformation is:

J_W = ∣∂U / ∂W∣ = ∣ 1/W∣ = 1/ w

Therefore, the density function for the ratio W is:

`f_W` (w) =
`\int\limits^ \infty _w {5ue^(-u)} \, du` =
`(5ue^(-w))/(w^2)` for w>0

So, the density function for the ratio of the lifetimes of the two types of components is
`(5ue^(-w))/(w^2)`, where w is the ratio of the lifetime of the second component to the first.