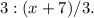Question

Simplify completely the expression (x² + 12x + 35) / (3x + 15).

1) (x² + 21) / 3
2) (x² + 11) / 3
3) (x + 7) / 3
4) (x + 5) / 3

Answer 1

The expression
`(x² + 12x + 35) / (3x + 15)` simplifies to
`(x + 7) / 3` after factoring and canceling out common terms; therefore, the correct answer is option
`3.`

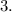
Step-by-step explanation:

To simplify completely the expression
`(x² + 12x + 35) / (3x + 15),`

we should factor both the numerator and the denominator, if possible.

The numerator factors to
`(x + 5)(x + 7)` because
`x² + 12x + 35` is a quadratic expression that can be factored into two binomials whose product gives the original quadratic.

On the other hand, the denominator,
`3x + 15,` can be simplified by factoring out the common factor of 3,

which gives us
`3(x + 5).`

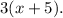
After factoring we have the following expression:

`((x + 5)(x + 7)) / (3(x + 5)).`

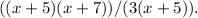
We notice that
`(x + 5)` appears in both the numerator and the denominator, so we can cancel this term out.

After canceling, the expression simplifies to
`(x + 7) / 3.`

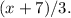
Therefore, the correctly simplified expression is option
`3: (x + 7) / 3.`