Question

What is the equation of the line passing through the points (2,1) and (5,-8)?

Answer 1

y = - 3x + 7

Step-by-step explanation:

the equatio of a line in slope- intercept form is

y = mx + c ( m is the slope and c the y- intercept )

calculate the slope m, using the slope formula

m =
`(y_(2)-y_(1) )/(x_(2)-x_(1) )`

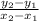
let (x₁, y₁ ) = (2, 1 ) and (x₂, y₂ ) = (5, - 8 )

substitute these values into the formula for m

m =
`(-8-1)/(5-2)` =
`(-9)/(3)` = - 3 , then

y = - 3x + c ← is the partial equation

to find c, substitute either of the 2 points into the partial equation

using (2, 1 ) for x and y in the partial equation

1 = - 3(2) + c = - 6 + c ( add 6 to both sides )

7 = c

y = - 3x + 7 ← equation of line

Answer 2

The equation of the line passing through the points (2,1) and (5,-8) is y = -3x + 7.

Step-by-step explanation:

The equation of the line passing through the points (2,1) and (5,-8) can be found using the slope-intercept form, y = mx + b.

Step 1: Find the slope (m) of the line using the formula m = (y2 - y1) / (x2 - x1). So, m = (-8 - 1) / (5 - 2) = -9/3 = -3.

Step 2: Use one of the given points and the previously found slope to substitute into the slope-intercept form and solve for the y-intercept (b). We'll use the point (2,1).

1 = -3(2) + b, which simplifies to 1 = -6 + b. Adding 6 to both sides of the equation gives us b = 7.

Step 3: Substitute the slope and y-intercept into the equation of the line, giving us y = -3x + 7.