Answer: 26.457 cm
Step-by-step explanation:
First, the height of an equilateral triangle of side length a is:
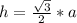
In this case, the side-length of the triangles is a = 10cm, then the height will be:

Now we can think on a triangle rectangle such that:
Point A and point F are vertexes of the triangle.
The line we want to find, the line from A to F, is the hypotenuse.
One cathetus is a the height of the triangle, that comes down from point A, the length of this cathetus is 8.66cm
The other cathetus is equal to half the side length of the triangles (5cm) plus the segments BD and DF (of 10cm each)
Then the length of this cathetus is 10cm + 10cm + 5cm = 25cm
Now, we can remember the Pythagorean theorem.
If H is the hypotenuse, and A and B are the catheti, then we have:
A^2 + B^2 = H^2
In this case, we know the length of both cathetus, and want to know the length of the hypotenuse, then:
(25cm)^2 + (8.66cm)^2 = H^2
√( (25cm)^2 + (8.66cm)^2) = H = 26.457 cm
Then the line from A to F is 26.457 cm