Question

Current assets $ 1,600

Net fixed assets 2,400
Total assets $ 4,000

Accounts payable and accruals $ 800
Short-term debt 100
Long-term debt 725
Preferred stock (15,000 shares) 475
Common stock (40,000 shares) 925
Retained earnings 975
Total common equity $ 1,900
Total liabilities and equity $ 4,000
Skye's earnings per share last year were $3.75. The common stock sells for $50.00, last year's dividend (D0) was $2.85, and a flotation cost of 9% would be required to sell new common stock. Security analysts are projecting that the common dividend will grow at an annual rate of 10%. Skye's preferred stock pays a dividend of $3.85 per share, and its preferred stock sells for $35.00 per share. The firm's before-tax cost of debt is 9%, and its marginal tax rate is 25%. The firm's currently outstanding 9% annual coupon rate, long-term debt sells at par value. The market risk premium is 5%, the risk-free rate is 6%, and Skye's beta is 1.786. The firm's total debt, which is the sum of the company's short-term debt and long-term debt, equals $0.825 million.

Answer 1

a. After-tax cost of debt is 6.75%, cost of preferred stock is 11%, cost of retained earnings is 2.4%, and cost of new common stock is 8.24%.

b. Cost of common equity from retained earnings using CAPM is 14.93%.

c. Cost of new common stock based on CAPM is 2.4%.

d. WACC is 8.89%.

a.

`\[ \text{After-tax cost of debt:} \]`

The cost of debt is given as 9%. Since it is before-tax, the after-tax cost of debt can be calculated as follows:

`\[ \text{After-tax cost of debt} = \text{Cost of debt} * (1 - \text{Tax rate}) \]`

`\[ \text{After-tax cost of debt} = 0.09 * (1 - 0.25) = 0.0675 \text{ or } 6.75\% \]`

`\[ \text{Cost of preferred stock:} \]`

`\[ \text{Cost of preferred stock} = \frac{\text{Dividend per share}}{\text{Price per share}} \]`

`\[ \text{Cost of preferred stock} = (3.85)/(35.00) \approx 0.11 \text{ or } 11\% \]`

`\[ \text{Cost of retained earnings:} \]`

Since Skye's earnings per share (EPS) last year were $3.75, the cost of retained earnings is the dividend payout ratio times the EPS:

`\[ \text{Dividend Payout Ratio} = \frac{\text{Dividends}}{\text{Earnings per share}} \]`

`\[ \text{Dividend Payout Ratio} = (2.85)/(3.75) \approx 0.76 \text{ or } 76\% \]`

`\[ \text{Cost of retained earnings} = \text{Retention Ratio} * \text{Return on Equity (ROE)} \]`

`\[ \text{Cost of retained earnings} = 0.24 * 0.10 \approx 0.024 \text{ or } 2.4\% \]`

`\[ \text{Cost of new common stock:} \]`

The cost of new common stock is calculated using the DCF method, considering the flotation cost:

`\[ \text{Cost of new common stock} = \frac{\text{Dividends per share}}{\text{Net issuing price}} \]`

`\[ \text{Flotation cost} = 0.09 * 50.00 = 4.50 \]`

`\[ \text{Net issuing price} = 50.00 - 4.50 = 45.50 \]`

`\[ \text{Cost of new common stock} = (3.75)/(45.50) \approx 0.0824 \text{ or } 8.24\% \]`

b.

`\[ \text{Cost of common equity from retained earnings using the CAPM method:} \]`

`\[ \text{Cost of common equity from retained earnings (re)} = \text{Risk-free rate} + (\text{Beta} * \text{Market risk premium}) \]`

`\[ \text{Cost of common equity from retained earnings (re)} = 0.06 + (1.786 * 0.05) = 0.1493 \text{ or } 14.93\% \]`

c.

`\[ \text{Cost of new common stock based on the CAPM:} \]`

Using the CAPM, the cost of new common stock is given by:

`\[ \text{Cost of new common stock (rs)} = \text{Risk-free rate} + (\text{Beta} * \text{Market risk premium}) \]`

`\[ \text{Cost of new common stock (rs)} = 0.06 + (1.786 * 0.05) = 0.1493 \text{ or } 14.93\% \]`

The difference between the cost of retained earnings (
`\(r_e\)`) and the cost of new common stock (
`\(r_s\)`) is:

`\[ \text{Difference} = r_e - r_s \]`

`\[ \text{Difference} = 0.024 - 0.1493 = -0.1253 \text{ or } -12.53\% \]`

The cost of new common stock based on the CAPM is
`\(r_s + \text{Difference}\):`

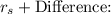
`\[ \text{Cost of new common stock based on the CAPM} = 0.1493 - 0.1253 = 0.024 \text{ or } 2.4\% \]`

d.

`\[ \text{Weighted Average Cost of Capital (WACC):} \]`

`\[\text{WACC} = \text{Weight of debt} * \text{Cost of debt} * (1 - \text{Tax rate}) + \text{Weight of preferred stock} * \text{Cost of preferred stock} + \text{Weight of common equity} * \text{Cost of common equity}\]`

`\[ \text{Weight of debt} = \frac{\text{Total debt}}{\text{Total liabilities and equity}} \]`

`\[ \text{Weight of debt} = (0.825)/(4.000) = 0.20625 \]`

`\[ \text{Weight of preferred stock} = \frac{\text{Market value of preferred stock}}{\text{Total liabilities and equity}} \]`

`\[ \text{Weight of preferred stock} = (15,000 * 35.00)/(4,000) = 0.328125 \]`

`\[ \text{Weight of common equity} = \frac{\text{Market value of common equity}}{\text{Total liabilities and equity}} \]`

`\[ \text{Weight of common equity} = ((40,000 * 50.00) + 975)/(4,000) = 0.465625 \]`

`\[\text{WACC} = (0.20625 * 0.0675) + (0.328125 * 0.11) + (0.465625 * 0.024)\]`

`\[ \text{WACC} \approx 0.0417 + 0.03605 + 0.01115 \approx 0.0889 \text{ or } 8.89\%\]`

So, the WACC assuming only retained earnings for equity is 8.89%, and the WACC if new common stock is issued is also 8.89% (using the simple average).