Question

Find three ordered pairs that are solutions to the system of equations

y=1/2x-2
(0,2)
(2,-1)
(4,0)
(0,-2)
(2,0)
(0,4)

Answer 1

Answer: (0,-2) (0,4)

Explanation:

I searched up y=1/2x-2 on desmos (graphing calculator) and I could only find 2 ordered pairs.

Answer 2

(2, -1), (4, 0), and (0, -2)

Explanation:

To check whether the given ordered pairs are solutions to the system of equations
`\sf y = (1)/(2)x - 2`, substitute the x and y values into the equation and see if the equality holds.

Let's evaluate each pair:

1. (0, 2):

`\sf y = (1)/(2)(0) - 2 = -2`

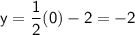
So, the coordinates (0, 2) are not a solution.

2. (2, -1):

`\sf y = (1)/(2)(2) - 2 = -1`

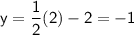
This satisfies the equation, so (2, -1) is a solution.

3. (4, 0):

`\sf y = (1)/(2)(4) - 2 = 0`

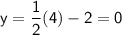
This satisfies the equation, so (4, 0) is a solution.

4. (0, -2):

`\sf y = (1)/(2)(0) - 2 = -2`

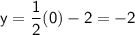
This satisfies the equation, so (0, -2) is a solution.

5. (2, 0):

`\sf y = (1)/(2)(2) - 2 = -1`

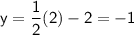
This satisfies the equation, so (2, 0) is not a solution.

6. (0, 4):

`\sf y = (1)/(2)(0) - 2 = -2`

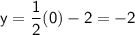
So, the coordinates (0, 4) are not a solution.

Therefore, the ordered pairs (2, -1), (4, 0), and (0, -2) are solutions to the system of equations.