Question

The mean diameter of the rim of Honda tires is 16 inches. Assume that the standard deviation of diameter of the rims is 0.3 inches. For quality control purposes, the diameter of the rims of 9 tires is measured every hour. The manager applies the rule that if the mean of diameter of a rim is greater or equal to 16.25, and lesser or equal to 15.75, the manufacturing should be stopped. If the diameter is between 15.75 and 16.25, the manufacturing process is not to be disturbed. a. Calculate the probability of stopping the manufacturing when the sample mean is 16 inches. b. Calculate the probability of stopping the manufacturing in case the mean is shifted to 16.05 inches. c. Calculate the probability of not disturbing the manufacturing if mean shifts to 16.25 inches.

Answer 1

a. 0.0124 = 1.24% probability of stopping the manufacturing when the sample mean is 16 inches.

b. 0.0241 = 2.41% probability of stopping the manufacturing in case the mean is shifted to 16.05 inches.

c. 0.5 = 50% probability of not disturbing the manufacturing if mean shifts to 16.25 inches.

Explanation:

To solve this question, we need to understand the normal probability distribution and the central limit theorem.

Normal probability distribution

When the distribution is normal, we use the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

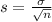Assume that the standard deviation of diameter of the rims is 0.3 inches. Samples of 9.

This means that
`\sigma = 0.3, n = 9, s = (0.3)/(√(9)) = 0.1`

a. Calculate the probability of stopping the manufacturing when the sample mean is 16 inches.

Here we have
`\mu = 16`

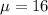
Higher than 16.25:

This is 1 subtracted by the pvalue of Z when X = 16.25. So

`Z = (X - \mu)/(\sigma)`

By the Central Limit Theorem

`Z = (X - \mu)/(s)`

`Z = (16.25 - 16)/(0.1)`

`Z = 2.5`

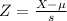
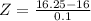
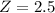
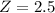`Z = 2.5` has a pvalue of 0.9938

1 - 0.9938 = 0.0062

Lower than 15.75:

This is the pvalue of Z when X = 15.75. So

`Z = (X - \mu)/(s)`

`Z = (15.75 - 16)/(0.1)`

`Z = -2.5`

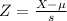
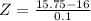
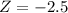
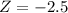`Z = -2.5` has a pvalue of 0.0062

Probability of stopping:

2*0.0062 = 0.0124

0.0124 = 1.24% probability of stopping the manufacturing when the sample mean is 16 inches.

b. Calculate the probability of stopping the manufacturing in case the mean is shifted to 16.05 inches.

Here we have
`\mu = 16.05`

Higher than 16.25:

`Z = (X - \mu)/(s)`

`Z = (16.25 - 16.05)/(0.1)`

`Z = 2`

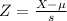
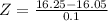
`Z = 2` has a pvalue of 0.9772

1 - 0.9772 = 0.0228

Lower than 15.75:

This is the pvalue of Z when X = 15.75. So

`Z = (X - \mu)/(s)`

`Z = (15.75 - 16.05)/(0.1)`

`Z = -3`

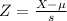
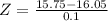
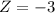
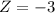`Z = -3` has a pvalue of 0.0013

Probability of stopping:

0.0228 + 0.0013 = 0.0241

0.0241 = 2.41% probability of stopping the manufacturing in case the mean is shifted to 16.05 inches.

c. Calculate the probability of not disturbing the manufacturing if mean shifts to 16.25 inches.

Between 16.25 and 15.75 with
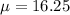`\mu = 16.25`. This is the pvalue of Z when X = 16.25 subtracted by the pvalue of Z when X = 15.75.

X = 16.25

`Z = (X - \mu)/(s)`

`Z = (16.25 - 16.25)/(0.1)`

`Z = 0`

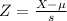
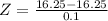
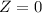
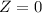`Z = 0` has a pvalue of 0.5

X = 15.75

`Z = (X - \mu)/(s)`

`Z = (15.75 - 16.25)/(0.1)`

`Z = -5`

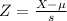
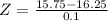
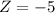
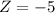`Z = -5` has a pvalue of 0

0.5 - 0 = 0.5

0.5 = 50% probability of not disturbing the manufacturing if mean shifts to 16.25 inches.