Question

Use the graph of logarithmic function f(x) f ( x ) to answer the question. A graph of an increasing log function that passes through (1, 0) and has a vertical asymptote at x equals 0. © 2017 StrongMind. Created using GeoGebra. What is the domain and range of the function? Match the domain and range with the corresponding set of points.

Answer 1

The logarithmic function f(x) is increasing, passing through (1, 0), with a vertical asymptote at x = 0. Its domain is
`\( (0, \infty) \)`, and the range is
`\( (-\infty, \infty) \).`

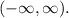
The given information describes a logarithmic function f(x) that is increasing, passes through the point (1, 0), and has a vertical asymptote at x = 0.

Domain:

The domain of a logarithmic function with a vertical asymptote at x = 0 is
`\( (0, \infty) \)` because logarithmic functions are undefined for non-positive values.

Range:

The range of an increasing logarithmic function is
`\( (-\infty, \infty) \)` because as x increases, the function values also increase without bound.

So, to match with the corresponding set of points:

- Domain: (0, ∞)

- Range: (-∞, ∞)

These sets represent the valid input values (domain) and the possible output values (range) for the given logarithmic function.